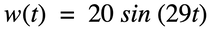Objective: Apply the sine function in a real world problem.
Sand Castles:
Ande loves to build elaborate sand castles at the beach. In fact, he is so skilled at making them they often take a long time to build. But sadly, the tides come in and demolish Ande’s work of art.
Ande loves to build elaborate sand castles at the beach. In fact, he is so skilled at making them they often take a long time to build. But sadly, the tides come in and demolish Ande’s work of art.
Since Ande has a mathematical mind he has spent scrupulous time modeling the tides at the beach. According to his analysis, the equation of the tides goes as follows:
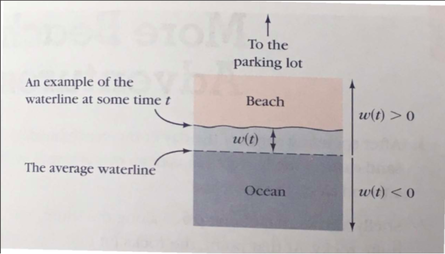
- w(t)represents the waterline above and below its average position
- t represents time
- The function represents the waterline for a 24 hour period
Your Task:
Ande needs to be closer to the waterline to maximize his skills building a sand castle.
Ande needs to be closer to the waterline to maximize his skills building a sand castle.
- If he builds 10ft below the waterline, what time should he come to the beach to maximize building time?
- How is the tide changing over time, what is the average rate of change of the tide?